Table of Contents
NDA SETS PREVIOUS YEAR QUESTIONS
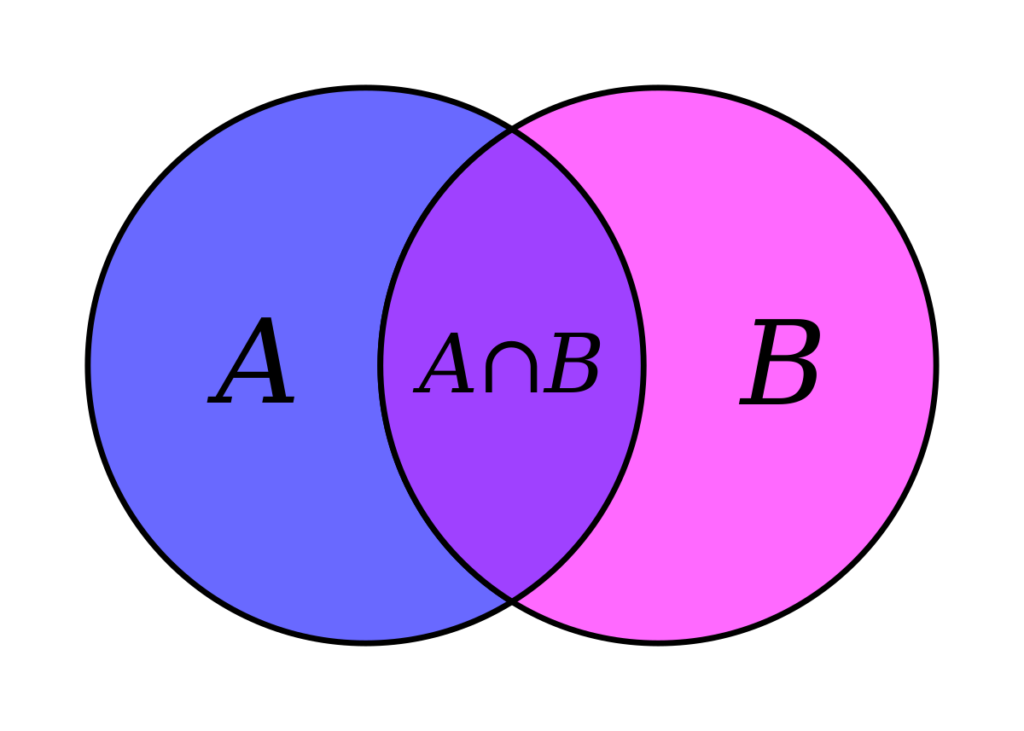
Table of Contents

INTRODUCTION
This Practice Set for All Competitive Exams Make Important Roll For NDA, NAVY, AIRFORCE, and SAT. Important Practice Set for All Competitive Exams is Easy to Learn and Questions are Important for exam preparations. This topic is very important for above exams because every year approx 3-4 question are asking in each paper of NDA.
Set and their representations
A set is a well-defined collection of objects.
There are two methods of representing a set
(i) Roaster or tabular form (ii) Set builder form
The empty set A set which does not contain any element is called the empty set or the void set or null set and is denoted by { } or φ.
NDA SETS PREVIOUS YEAR QUESTIONS
Singleton Set
A set which does not contain any element is called the Singleton set .
Finite and infinite sets A set which consists of a finite number of elements is called a finite set otherwise, the set is called an infinite set.
Cardinal number The number of elements in finite set is represented by n(A), known as Cardinal number.
Subsets A set A is said to be a subset of set B if every element of A is also an element of B. In symbols we write A ⊂ B if a ∈ A ⇒ a ∈ B.
We observe that N ⊂ Z ⊂ Q ⊂ R, T ⊂ R, Q ⊄ T, N ⊄ T
Note: The set is also a subset of itself.
If A is not a subset of B, then it is denoted as A⊄B.
If A is a set with n(A) = m, then the number of subsets of A are 2m and the number of proper subsets of A are 2m -1.
Equivalent set
If the number of elements is the same for two different sets, then they are called equivalent sets. The order of sets does not matter here. It is represented as:
n(A) = n(B)
where A and B are two different sets with the same number of elements.
Equal sets
Given two sets A and B, if every elements of A is also an element of B and if every element of B is also an element of A, then the sets A and B are said to
be equal. The two equal sets will have exactly the same elements.
Intervals as subsets of R Let a, b ∈ R and a < b. Then
(a) An open interval denoted by (a, b) is the set of real numbers {x : a < x < b}
(b) A closed interval denoted by [a, b] is the set of real numbers {x : a ≤ x ≤ b)
(c) Intervals closed at one end and open at the other are given by
[a, b) = {x : a ≤ x < b} (a, b] = {x : a < x ≤ b}
Power set The collection of all subsets of a set A is called the power set of A.
NDA SETS PREVIOUS YEAR QUESTIONS
It is denoted by P(A). If the number of elements in A = n , i.e., n(A) = n, then the number of elements in P(A) = 2n.
Universal set This is a basic set; in a particular context whose elements and subsets are relevant to that particular context. For example, for the set of vowels in English alphabet, the universal set can be the set of all alphabets in English. Universal set is denoted by U.
Venn diagrams Venn Diagrams are the diagrams which represent the relationship between sets. For example, the set of natural numbers is a subset of set of whole numbers which is a subset of integers. We can represent this relationship through Venn diagram in the following way
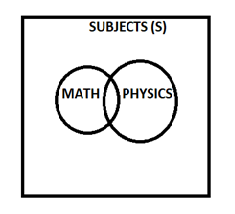
OPERATIONS ON SETS
UNION OF TWO SETS
The union of two sets A and B, written as A ∪ B (read as “A union B”), is the set consisting of all the elements which are either in A or in B or in both Thus,
A ∪ B = {x : x ∈ A or x ∈ B}
Clearly, x ∈ A ∪ B à x ∈ A or x ∈ B, and
xÏ A ∪ B à x Ï A and x Ï B.
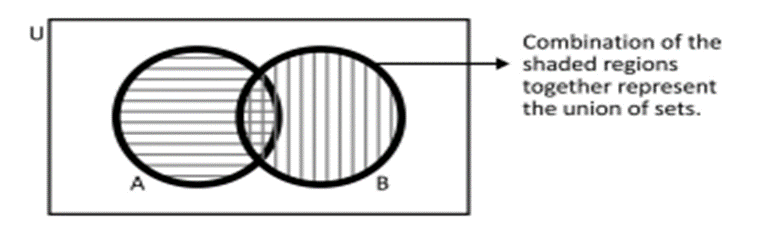
For example, if A={a, b, c, d} and B= {c, d, e, f}, then AUB = {a, b, c, d, e, f}
NDA SETS PREVIOUS YEAR QUESTIONS
Intersection of Two sets
The intersection of two sets A and B, written as A ∩ B (read as ‘A’ intersection ‘B’) is the set consisting of all the common elements of A and B. Thus,
A ∩ B = {x : x ∈ A and x ∈ B}
Clearly, x ∈ A∩B →x ∈ A and x ∈ B, and x ÏA∩B à xÏA or x ÏB.
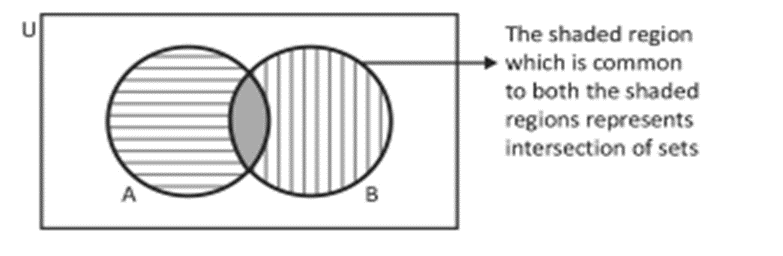
For example, if A = {a, b, c, d) and B = {c, d, e, f}, then A ∩ B = {c, d}.
Disjoint Sets
Two sets A and B are said to be disjoint, if A ∩ B = ɸ , i.e. A and B have no element in common.
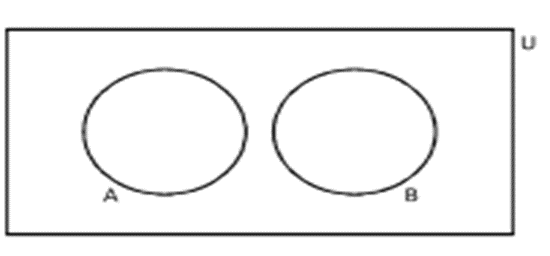
For example, if A = {1, 3, 5} and B = {2, 4, 6}, then A ∩ B = ɸ , so A and B are disjoint sets
NDA SETS PREVIOUS YEAR QUESTIONS
Difference of Two Sets
If A and B are two sets, then their difference A – B is defined as :
A – B = {x : x ∈ A and x Ï B}.
Similarly, B – A = {x : x ∈ B and x ÏA }.
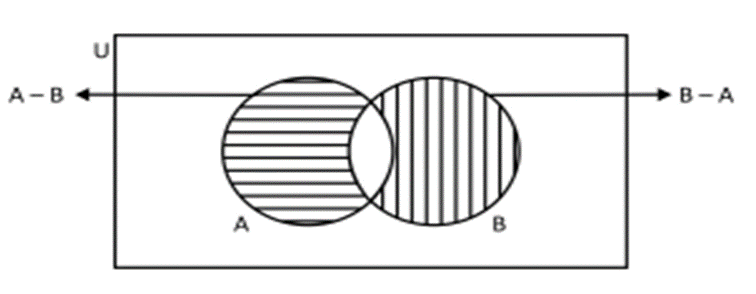
For example, if A = {1, 2, 3, 4, 5} and B = {1, 3, 5, 7, 9} then A – B = {2, 4} and B – A = {7, 9}.
Important Results
(a) A – B ≠ B – A
(b) The sets A – B , B – A and A ∩ B are disjoint sets
(c) A – B ⊆ A and B – A ⊆ B
(d) A – ɸ = A and A – A = ɸ
Symmetric Difference of Two Sets
The symmetric difference of two sets A and B , denoted by A ∆ B, is defined as
A ∆ B = (A – B) ∪ (B – A).
For example, if A = {1, 2, 3, 4, 5} and B = {1, 3, 5, 7, 9}
then A ∆ B = (A – B) ∪ (B – A) = {2, 4} ∪ {7, 9} = {2, 4, 7, 9}.
Complement of a Set

5. ALGEBRA OF SETS
1. For any set A , we have
a) A ∪ A = A b) A ∩ A = A
2. For any set A, we have
a) A ∪ ɸ = A b) A ∩ = ɸ = ɸ
c) A ∪ U = U d) A ∩ U = A
3. For any two sets A and B, we have
a) A ∪ B = B ∪ A b) A ∩ B = B ∩ A
4. For any three sets A, B and C, we have
a) A ∪ (B ∪ C) = (A ∪ B) ∪ C b) A ∩ (B ∩ C) = (A ∩ B) ∩ C
5. For any three sets A, B and C, we have
a) A ∪ (B ∩ C) = (A ∪ B) ∩ (A ∪ C)
b) A ∩ (B ∪ C) = (A ∩ B) ∪ (A ∩ C)
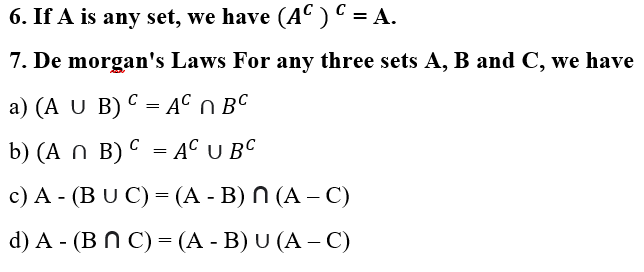
NDA( 2021)Paper 2
1. The union over intersection of sets is distributive.
2. The complement of union of two sets is equal to intersection of their complements.
3. If the difference of two sets is equal to empty set, then the two sets must be equal.
Which of the above statements are correct?
(a) 1 and 2 only
(b) 2 and 3 only
(c) 1 and 3 only
(d) 1, 2 and 3
Let S = (X –Y) ∪ Z. How many proper subsets does S have?
(a) 255
(b) 256
(c) 1023
(d) 1024
(a) 324
(b) 364
(c) 384
(d) 400
NDA( 2021)Paper 1
(a) A and B should be square matrices of same order.
(b) Either A or B should be a square matrix
(c) Both A and B should be of same order.
(d) Orders of A and B need not be the same.
1. The null set is a subset of every set.
2. Every set is in a subset of itself.
3. If a set has 10 elements, then its power set will have 1024 elements.
Which of the above statements are correct?
(a) 1 and 2 only
(b) 2 and 3 only
(c) 1 and 3 only
(d) 1, 2 and 3
(a) 36 °
(b) 72°
(c) 120 °
(d) 168°
1. A = (1, 3, 5) and B = {2, 4, 7} are equivalent sets.
2. A = (1, 5, 9) and B = (1, 5, 5, 9, 9) are equal sets.
Which of the above statements is / are correct?
(a) 1 only
(b) 2 only
(c) Both 1 and 2
(d) Neither 1 nor 2
NDA( 2020)
Directions for the following three (03) items:
Consider the following Venn diagram, where X, Y and Z are three sets. Let the number of elements in Z be denoted by n(Z) which is equal to 90.
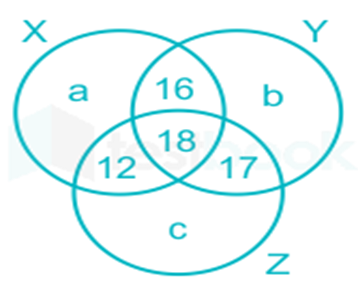
(a) 18 (b) 19 (c) 21 (d) 23
(a) a + b + 43 (b) a + b + 63
(c) a + b + 96 (d) a + b + 106
(a) p + b + 60
(b) p + b + 40
(c) p + a + 60
(d) p + a + 40
NDA 2019 Paper1
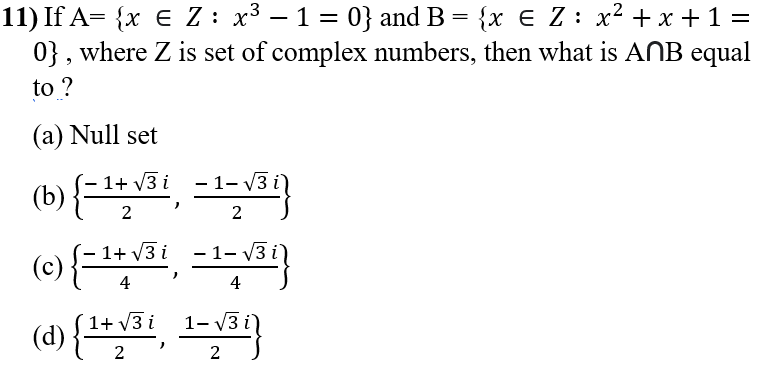

1. A ⊂ C à (A ∩ B) ⊂ (C ∩ B) (A ∪ B) ⊂ (C ∩ B)
2. (A ∩ B) ⊂ (C ∩ B) for all sets B à A ⊂ C
3. (A ∪ B) ⊂ (C ∪ B) for all sets B à A ⊂ C
Which of the above statements are correct?
(a) 1 and 2 only (b) 2 and 3 only
(c) 1 and 3 only (d) 1, 2 and 3
In a school, all the students play at least one three indoor games – chess, carrom and table tennis. 60 play chess, 50 play table tennis, 48 play carrom, 12 play chess and carrom, 15 play carrom and table tennis, 20 play table tennis and chess.
(a) 123
(b) 111
(c) 95
(d) 63
(a) 111
(b) 123
(c) 125
(d) 135
NDA 2019 Paper 2
(a) A ∪ (A ∩ B) = A ∪ B
(b) A ∩ (A ∪ B) = A
(c) (A ∩ B) ∪ C = (A ∪ C) ∩ (B ∪ C)
(d) (A ∪ B) ∩ C = (A ∩ C) ∪ (B ∩ C)
(a) 3 (b) 6 (c) 8 (d) 9
(a) Ø
(b) {1}
(c) {2}
(d) {1, 2}
(a) A = {x | x > a} and B = {x | x > b}
(b) A = {x | x < a} and B = {x | x > b}
(c) A = {x | x < a} and B = {x | x < b}
(d) A = {x | x > a} and B = {x | x < b}
(a) 10
(b) 20
(c) 512
(d) 1024
NDA 2018 Paper 1
(a) (A ∪ B’) – (A ∩ B’)
(b) (A ∪ B) – (A ∩ B)
(c) (A ∪ B) – (A ∩ B)
(d) (A’ ∪ B’) – (A’ ∩ B’)
NDA SETS PREVIOUS YEAR QUESTIONS
Consider the information given below and answer the two items (02) that follow :
In a class, 54 students are good in Hindi only. 63 students are good in Mathematics only and 41 students are good in English only. There are 18 students who are good in both Hindi and Mathematics. 10 students are good in all three subjects.
(a) 99
(b) 107
(c) 125
(d) 130
(a) 18
(b) 12
(c) 10
(d) 8
(a) 40
(b) 45
(c) 50
(d) 55
NDA 2018 Paper 2
Consider the information given below and answer the two (02) items that follow:
A survey was conducted 300 students It was found that 125 students like to play cricket, 145 students like to play football and 90 students like to play tennis 32 students like to play exactly two games out of the three Games.
(a) 14 (b) 21 (c) 28 (d) 35
(a) 196 (b) 228 (c) 254 (d) 268
2017 NDA Paper 1
(a) The relation is an equivalent relation
(b) The relation is not reflexive but it is symmetric and transitive
(c) The relation is not symmetric but it is reflexive and transitive
(d) The relation is not transitive but it is reflexive and symmetric
(a) 45 (b) 120 (c) 165 (d) 330
1. (A – B) ∪ B = A
2. (A – B) ∪ A = A
3. (A – B) Ç B = ɸ
4. A ⊆ B ð$ A ∪ B = B
Which of the above are correct?
(a) 1, 2 and 3
(b) 2, 3 and 4
(c) 1, 3 and 4
(d) 1, 2 and 4
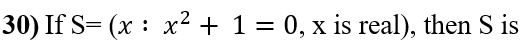
(a) {- 1} (b) {0} (c) {1} (d) an empty set
NDA 2017 Paper -2
the set E – (E – (E – (E – (E – A)))) is same as the set
(a) B’ ∪ C’
(b) B ∪ C
(c) B’ ∩ C’
(d) B ∩ C
(a) A
(b) B
(c) C
(d) {x : x is a multiple of 100}
NDA 2016 Paper -2
Select the correct answer using the code given below :
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| d | c | a | C | d | b | c | c | d | a |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 |
| c | a | c | b | b | a | d | c | b | d |
| 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | 30 |
| c | c | d | b | a | c | a | c | b | d |
| 31 | 32 | 33 | 34 | 35 | |||||
| c | c | b | c | d |
ALSO VISIT : QUSETIONS ON SETS
DOWNLOAD MOBILE APPLICATION TO LEARN MORE: NDA SETS PREVIOUS YEAR QUESTIONS